Journal of Physical Chemistry & Biophysics
Open Access
ISSN: 2161-0398
ISSN: 2161-0398
Research Article - (2016) Volume 6, Issue 2
In thermodynamics entropy Std is an extensive state function. Its derivation by statistical mechanics following Boltzmann and Gibbs with the famous formula S=kBlnW for a micro-canonical ensemble with N particles, kB the Boltzmann constant, and W the number of accessible micro-states is however in general not extensive unless the Stirling approximation given by lnN! – NlnN + N is used. Furthermore, at the thermodynamic limit with the number of particles N→∞ at constant density the Stirling approximation can not be used to show extensivity because limN→∞ (lnN! – NlnN + N)=∞. Hence, the Boltzmann entropy S as shown here for the ideal gas is neither for a small system with N particles nor at the thermodynamic limit extensive. Thus, if strict extensivity for the entropy is requested the claim of statistical mechanics that the Boltzmann entropy is a microscopic description of its thermodynamic analog is challenged.
<Keywords: Entropy; Boltzmann; Stirling formula; Ideal gas
Thermodynamics describes a physical system in a given state from a macroscopic point of view with extensive and intensive state functions. Extensive state functions such as the volume V, the number of particles N, and the entropy Std are proportional to the size of the system (i.e., the amount of material) while intensive state functions such as the temperature T and the pressure p do not scale with the system size. These definitions may be best described by the doubling of a system by merging the original system with an equal system. Since in the absence of surface effects and interacting effects between the two subsystems the extensive state functions are proportional to the size of the system they will double, while the intensive state functions will not change since they are independent of the system size. Obviously, the statistical mechanics-based microscopic descriptions of these thermodynamic quantities must also obey their extensive or intensive character at least under the conditions of the thermodynamic limit defined by N→∞ (and at constant density 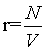 ), at which statistical mechanics is believed to approximate thermodynamics [1]. Thus, the Boltzmann entropy S, which is at the heart of statistical mechanics, must be extensive at least at the thermodynamic limit and under idealized conditions such as absence of surface effects and interacting effects of the two subsystems as typically exemplified by the ideal gas. Following Gibbs and Boltzmann, the Boltzmann entropy S is a measure of the number of accessible micro-states of the system of interest in its thermodynamic equilibrium. For a micro canonical ensemble the Boltzmann entropy is given by S=kBlnW with kB the Boltzmann constant, and W the number of accessible micro-states [2,3]. Although it is usually stated in textbooks that for a system with N indistinguishable particles the Boltzmann entropy at the thermodynamic limit gets extensive after resolving the Gibbs paradox by scaling down the number of micro-states W by N! to take into account the indistinguishability of the particles, it is noteworthy to mention that this statistical mechanics approach requests the use of the Stirling approximation lnN!=NlnN – N [4-8]. It has been noted that the term N! within the natural logarithm poses potential issues on the Boltmann entropy [9-11] yielding alternative descriptions on entropy such as the “thermal” and “ configurational” views, [10] introducing the concept of an entropy density, [1,10,12,13] or even redefining the entropy [9,14]. Importantly, as we shall see for limN→∞ (lnN!=NlnN – N)=∞, [10,11,15] which means that at the thermodynamic limit this form of the Stirling approximation can not be used to prove the extensivity of the Boltzmann entropy. The consequence is that the Boltzmann entropy, as shown in the following for the ideal gas and other simple systems, is in general not extensive and thus not an extensive state function irrespective on the number of particles N present. The scaling down of W by N! solves in large extent the Gibbs paradox, but it does not bring extensivity even at the thermodynamic limit. Since however thermodynamics requests entropy to be an extensive state function, the Boltzmann entropy is challenged to be a microscopic description of its thermodynamic analog. This finding may revitalize the long standing objections on Boltzmann entropy such as the Loschmidt and Zermelo’ s reversal and reoccurrence objections [16,17] and may ask for alternative derivations [18,19].
), at which statistical mechanics is believed to approximate thermodynamics [1]. Thus, the Boltzmann entropy S, which is at the heart of statistical mechanics, must be extensive at least at the thermodynamic limit and under idealized conditions such as absence of surface effects and interacting effects of the two subsystems as typically exemplified by the ideal gas. Following Gibbs and Boltzmann, the Boltzmann entropy S is a measure of the number of accessible micro-states of the system of interest in its thermodynamic equilibrium. For a micro canonical ensemble the Boltzmann entropy is given by S=kBlnW with kB the Boltzmann constant, and W the number of accessible micro-states [2,3]. Although it is usually stated in textbooks that for a system with N indistinguishable particles the Boltzmann entropy at the thermodynamic limit gets extensive after resolving the Gibbs paradox by scaling down the number of micro-states W by N! to take into account the indistinguishability of the particles, it is noteworthy to mention that this statistical mechanics approach requests the use of the Stirling approximation lnN!=NlnN – N [4-8]. It has been noted that the term N! within the natural logarithm poses potential issues on the Boltmann entropy [9-11] yielding alternative descriptions on entropy such as the “thermal” and “ configurational” views, [10] introducing the concept of an entropy density, [1,10,12,13] or even redefining the entropy [9,14]. Importantly, as we shall see for limN→∞ (lnN!=NlnN – N)=∞, [10,11,15] which means that at the thermodynamic limit this form of the Stirling approximation can not be used to prove the extensivity of the Boltzmann entropy. The consequence is that the Boltzmann entropy, as shown in the following for the ideal gas and other simple systems, is in general not extensive and thus not an extensive state function irrespective on the number of particles N present. The scaling down of W by N! solves in large extent the Gibbs paradox, but it does not bring extensivity even at the thermodynamic limit. Since however thermodynamics requests entropy to be an extensive state function, the Boltzmann entropy is challenged to be a microscopic description of its thermodynamic analog. This finding may revitalize the long standing objections on Boltzmann entropy such as the Loschmidt and Zermelo’ s reversal and reoccurrence objections [16,17] and may ask for alternative derivations [18,19].
After a short review on various Stirling approximations and the Boltzmann entropy, text book examples of the Boltzmann entropies and their extensive character for an ideal gas, as well as a primitive real gas system are calculated with and without the use of two different Stirling approximations, followed by the calculation of the extensivity of the Boltzmann entropy for N particles distributed in M states, ended thereafter by a discussion and a conclusion.
The stirling approximations
The Stirling approximations [4,20] give an approximate value for the factorial function N!. The most often form used for statistical mechanics is given by
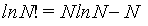 (1)
(1)
It corresponds to the first term of the Stirling’s series given by
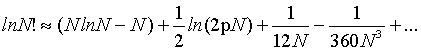 (2)
(2)
The factorial function N!. can also be described by the double inequality
 (3)
(3)
A better approximation is given by
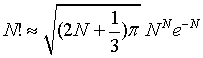 (4)
(4)
In the following some of the properties of Stirling’s approximation relevant for statistical mechanics are reviewed.
If the standard Stirling approximation equation 1 is used, for a N>100 the relative deviation of the right hand from the left hand side is smaller than 1% and decreases with larger N. While the relative deviation for limN→∞ →0 it is interesting to note that the absolute deviation is infinite, i.e., limN→∞ (lnN! – NlnN + N)=∞. This is numerically exemplified by Figure 1 and by the evaluation of the second term in the Stirling series, which can be used to estimate the deviation. For N∞ the term  increases logarithmically to infinity as observed in Figure 1.
increases logarithmically to infinity as observed in Figure 1.
In contrast, using the first two terms of the Stirling series
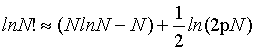 (5)
(5)
the error propagates with the third term in the Stirling series 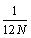 , and thus for N→∞ the absolute error is decreasing proportionally towards 0. It is therefore evident, that to approximate N! equations 4 or 5 should rather be used than equation 1.
, and thus for N→∞ the absolute error is decreasing proportionally towards 0. It is therefore evident, that to approximate N! equations 4 or 5 should rather be used than equation 1.
The Boltzmann entropy
The second law of thermodynamics states that the entropy Std (note, td is used for distinguishing the thermodynamic entropy from the Boltzmann entropy) of an isolated system increases over time until the system is in its thermodynamic equilibrium. The term entropy Std goes back to Clausius [21] who tried to design and understand “ heat engines”, which are cyclic machines for the conversion of heat Q into useful work, and found when averaged over many cycles that for an irreversible machine 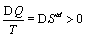 . The entropy is thereby an extensive state function as demonstrated by Carnot by studying the cyclic Carnot process [5]. Entropy exerts its presence also by being part of the total free energy. For example for an ideal gas the Gibbs free energy is given by G=U+pV – TStd with U the inner Energy, p the pressure, V the volume, and T the temperature.
. The entropy is thereby an extensive state function as demonstrated by Carnot by studying the cyclic Carnot process [5]. Entropy exerts its presence also by being part of the total free energy. For example for an ideal gas the Gibbs free energy is given by G=U+pV – TStd with U the inner Energy, p the pressure, V the volume, and T the temperature.
Following the systematic formulation of statistical mechanics by Gibbs and Boltzmann, the Boltzmann entropy S is a measure of the number of accessible micro-states of the system of interest in its thermodynamic equilibrium. For a micro canonical ensemble the Boltzmann entropy is given by
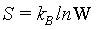 (6)
(6)
With kB the Boltzmann constant, and W the number of possible micro-states that describe the same macro-state. The statistical mechanics argument is sound, that a system has the tendency to evolve towards its most probable state, which is the equilibrium state.
The Boltzmann entropy is not strictly extensive in the most simple description of the ideal gas
Let us consider a diluted, ideal gas in two independent boxes (i=1,2) both with M sites and N undistinguishable particles each (M ? N). For simplicity each site may be occupied with more than one particle. It is the attempt to check for this system the extensivity of the Boltzmann entropy, i.e., whether the entropy of the two boxes together is the sum of the entropies of the individual boxes. Following Gibbs (after resolving the Gibb’s paradoxon)8 for each box the number of states possible is
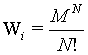 (7)
(7)
while for the two boxes together it is
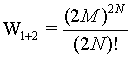 (8)
(8)
because the number of sites as well as the number of particles doubled. The term N! is necessary to account for the indistinguishability of the gas molecules. In order to show the extensive nature of the entropy, the entropy of the combined system given by S1+2 must be the sum of the entropies of the individual system 2*Si. With other words the following difference must be 0
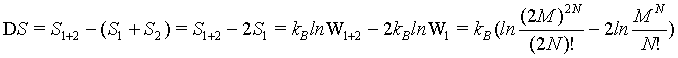 (9)
(9)
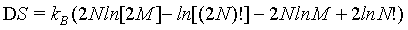 (10)
(10)
Using the Stirling approximation of equation 1 as is done usually in text books, the above expression can be simplified to
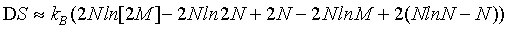 (11)
(11)
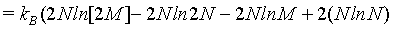
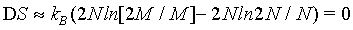 (12)
(12)
Hence, with the use of the simplified Stirling approximation (equation 1) the Boltzmann entropy is extensive. Actually with this derivation the Boltzmann entropy appears to be extensive irrespective to the number of particles N. The thermodynamic limit is however usually propagated in order to validate the use of the Stirling approximation. But, as shown above, for N→∞ the absolute error of the Stirling formula of equation 1 increases logarithmically to infinity, while only the relative error goes to 0. Since entropy is not a relative entity the Stirling approximation of equation 1 can not be used.
This finding is further demonstrated in the following. If the Stirling approximation is not used
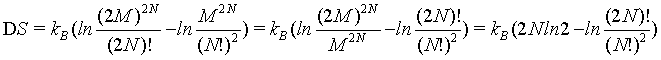 (13)
(13)
The quantitative plot of this equation (Figure 2) shows that DS increases with N highlighting that the Boltzmann entropy is not extensive neither for small number of particles N nor at the thermodynamic limit.
This problem can be demonstrated also by using the first two terms of the Stirling series (equation 5) for which the absolute error decreases proportionally with N and thus vanishes at the thermodynamic limit (see above). By using this version of the Stirling approximation the extensive nature of the Boltzmann entropy can be checked by
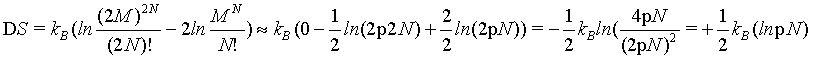 (14)
(14)
which at the thermodynamic limit
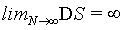 (15)
(15)
This finding disproves again that the Boltzmann entropy for the given example of an ideal gas with undistinguishable structureless particles is an extensive state function neither for small number of particles nor at the thermodynamic limit.
The finding does not change if again another approximation for N! is used (equation 4) with
 (16)
(16)
yielding
 (17)
(17)
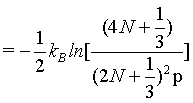 (18)
(18)
which at the thermodynamic limit (Table 1).
| 23.5cmApproximations for n! | Ω(M, N) | |
|---|---|---|
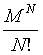 |
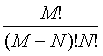 |
|
| n! | ∞ | ∞ for M ≠ N |
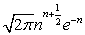 |
∞ | ∞ for M ≠ N |
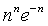 |
0 | 0 |
| nn | 0 | 0 |
Table 1: Limit of ΔS as N approaches infinity at constant ratio 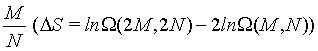
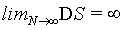 (19)
(19)
In summary, by calculating the Boltzmann entropy of the most simple model of an ideal gas it is concluded that the Boltzmann entropy is not strictly extensive, neither in a system with a small number of particles N nor at the thermodynamic limit.
The Boltzmann entropy is not strictly extensive for the “excluded-volume” gas model
Here, it is demonstrated that the Boltzmann entropy is also not extensive for a more accurate model of a gas that takes into account that two particles cannot overlap each other. This model is also called the “excluded-volume” model or the lattice gas model. We still consider two independent boxes (i=1,2) both with M sites and N undistinguishable particles in each box. The request of the excluded volume that no site can be occupied by more than one particle restricts the number of micro states as follows: The first particle can be in M sites, the second has only M – 1 choices to be located, the third M – 2 resulting in 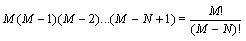 possible micro states. Together with the term N! which addresses the over counting due to the indistinguishability of the particles (i.e., the Gibbs paradox) the number of micro states that describe the macro state is given by
possible micro states. Together with the term N! which addresses the over counting due to the indistinguishability of the particles (i.e., the Gibbs paradox) the number of micro states that describe the macro state is given by
 (20)
(20)
while for the two boxes together the number of micro states is given by
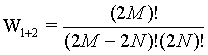 (21)
(21)
because the number of sites as well as the number of particles doubled.
In order to check the extensive nature of the Boltzmann entropy, the entropy difference between the combined system given by S1+2 and the sum of the entropies of the individual systems 2*Si is calculated:
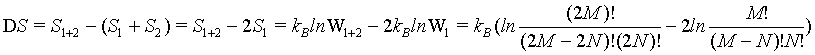 (22)
(22)
Rewriting equation 22 results in
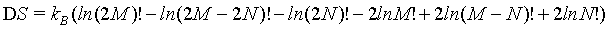 (23)
(23)
By applying the usually used Stirling formula of equation 1 to each of the terms we get
 (24)
(24)

and thus with the use of the Stirling approximation, the Boltzmann entropy appears to be extensive for any given particle number N.
However, if equation 22 is plotted (Figure 3) a numerical analysis thereof for limN∞ DS indicates DS→∞.
Similarly, by using the first two terms of the Stirling series (equation 5) equation 23
(DS=kB(ln(2M)! – ln(2M – 2N)! – ln(2N)! –2lnM!+2ln(M – N)!+2lnN) approximates for N≠ 0 to
 (25)
(25)
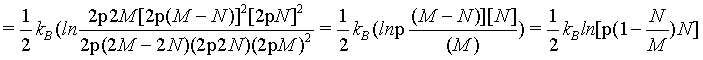 (26)
(26)
Since the density of the system 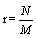 is an intensive state function and does therefore not change when increasing the system to the thermodynamic limit, for N ≠ M
is an intensive state function and does therefore not change when increasing the system to the thermodynamic limit, for N ≠ M
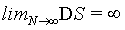 (27)
(27)
In summary, by deriving the Boltzmann entropy of the “excluded volume” gas model it is concluded that the Boltzmann entropy is not extensive, neither for a small number of particles N nor at the thermodynamic limit (Table 1).
The Boltzmann entropy is not strictly extensive for a N particles system distributed over M energy levels
Following Kondepudi [7] we consider a box i with N indistiuingishable particles whose energy can be any of the M possible values E1, E2,…., EM. At equilibrium the particles are distributed over the M energy levels such that there are N1, N2,…., NM with 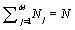 . The numbers of microstates that describe the same macrostate is then the number of distinct ways in which the particles can be distributed in the M states yielding
. The numbers of microstates that describe the same macrostate is then the number of distinct ways in which the particles can be distributed in the M states yielding
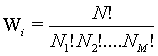 (28)
(28)
This number of microstates takes into account the number of indistinguishable permutations within the same energy level (i.e., Nj!, while the number of all permutations is N!. The Boltzmann entropy of this system is
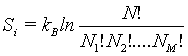 (29)
(29)
Following the approaches described above, to show the extensive nature of the Boltzmann entropy, the entropy S1+2 of a combined system of two independent boxes 1 and 2 must be the sum of the entropies of the individual subsystem 2*Si (For simplicity we chose system 1 and 2 are equal in nature). Thus,
 (30)
(30)
This equation can be simplified to
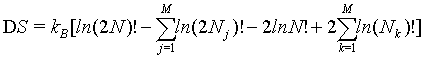 (31)
(31)
By applying the Stirling approximation of equation 1
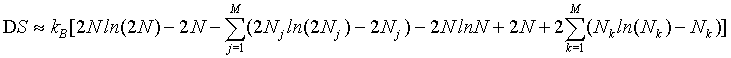 (32)
(32)
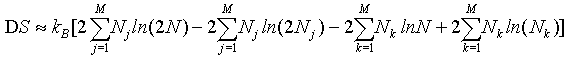 (33)
(33)
 (34)
(34)
Thus, by using the Stirling approximation of equation 1, the Boltzmann entropy of the given system is extensive. Furthermore, with the definition of the probability of occupying a state with energy Ej to be 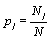 the Boltzmann entropy is given by the well known expression
the Boltzmann entropy is given by the well known expression 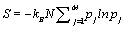 .
.
However, as we have seen above, the Stirling approximation of equation 5 should rather be used. By doing so:
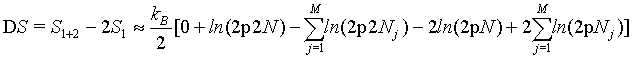 (35)
(35)
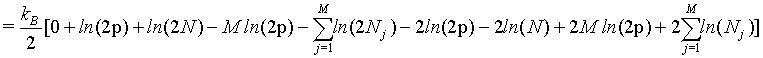 (36)
(36)
 (37)
(37)
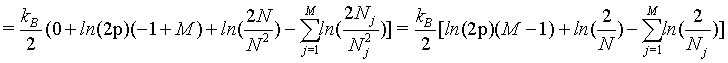 (38)
(38)

 (39)
(39)
which results in
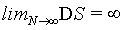 (40)
(40)
In summary, again and also in this example, the Boltzmann entropy is not extensive, neither at a small number of particles N nor at the thermodynamic limit.
The Boltzmann entropy is not extensive
Statistical mechanics has originated from the desire to obtain a microscopic understanding of macroscopic physics including in particular thermodynamics. Thermodynamic entities are thereby described (usually) at the thermodynamic limit. This includes the description of the thermodynamic entropy by the Boltzmann entropy. Unfortunately, as we have demonstrated above for three standard examples, the Boltzmann entropy is not extensive neither for a N particles system nor at the thermodynamic limit. Thus, if strict extensivity of the entropy is requested for thermodynamics, the Boltzmann entropy lacks an important property of its macroscopic analog. Actually, strict extensivity is a request because only with the extensive character of the entropy a mathematical description of thermodynamics is adequate. For example, if entropy is not strictly extensive, other extensive thermodynamic measures (such as the free energy or the volume) would loose their extensive character. Furthermore, there are pairs of intensive and extensive state functions such as T (temperature) and S as well as p (pressure) and V.
One may argue from a more practical point of view that the Boltzmann entropy is almost extensive and satisfies well the experimental data (i.e., if there is an error in the theoretical calculation it is too small to be measured). However, if the same argument would be applied to the mass of a system in classical mechanics it is the reviewer’s opinion that nobody would accept that the mass is only almost extensive. Actually, if the error made by the Stirling formula by calculating the entropy difference to a system with 1 mol particles is translated into its mass difference analog, an error in the order of 10-6 Joule is calculated. While in the case of mass such small differences would be detectable, it is unfortunately not possible to measure entropy with such an accuracy.
One may reason further that because at the thermodynamic limit N→ ∞, V→ ∞, the Boltzmann entropy S→ ∞, and DS→ ∞ for the examples given above, one should rather introduce an entropy density such as 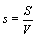 with V the volume of the system or equivalent an entropy per particle with
with V the volume of the system or equivalent an entropy per particle with 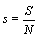 than the usually used Boltzmann entropy. Indeed, this approach resolves the problems about infinite values at the thermodynamic limit and
than the usually used Boltzmann entropy. Indeed, this approach resolves the problems about infinite values at the thermodynamic limit and  for the examples given above. However, by definition the entropy density is an intensive function and thus discussions about an extensive character must be regarded obsolete. Moreover, if the same argument would be applied to the mass of a system (introduced above), only the density of the system could be used anymore and the mass itself is regarded meaningless.
for the examples given above. However, by definition the entropy density is an intensive function and thus discussions about an extensive character must be regarded obsolete. Moreover, if the same argument would be applied to the mass of a system (introduced above), only the density of the system could be used anymore and the mass itself is regarded meaningless.
Another argument that one may put forward is that surface effects have not been accounted for in the present calculations and that they are of similar size as the error in made by using the Stirling formula. In counting the number of micro-states W surface effects are in the order of 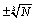 and the fluctuations
and the fluctuations 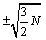 for a monoatomic ideal gas. The error made through the use of the Stirling formula is however
for a monoatomic ideal gas. The error made through the use of the Stirling formula is however 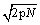 and is thus larger (and always positive).
and is thus larger (and always positive).
Overall it is summarized that based on the examples illustrated above the strict extensivity of the Boltzmann entropy is challenged.
It is the Stirling approximation that makes the Boltzmann entropy extensive
From a mathematical point of view the lack of extensivity of the Boltzmann entropy is attributed to the entity N! inside the ln. This entity originally introduced to resolve the Gibbs paradox is usually present in all model systems with indistinguishable particles whether the system under study is of discrete or continuous, of classical or quantum mechanical nature. This suggests that the Boltzmann entropy in general does not describe the thermodynamic entropy.
In this context we would like to note, that it is actually the now challenged use of Stirling’ s approximation of equation 1, which provides strict extensivity of the Boltzmann entropy for any number of states and particles. This fact has nothing to do with how good Stirling’ s approximation approaches the value of factorial. With the requirement for strict extensivity of the Boltzmann entropy given by W(kM,kN)=Wk(M,N) for any integer k with 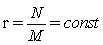 the Stirling approximation for the N factorial with NNe-N results in an extensive expression per se. In the first gas model described it results in the number of microstates with
the Stirling approximation for the N factorial with NNe-N results in an extensive expression per se. In the first gas model described it results in the number of microstates with 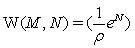 and in the second example to
and in the second example to 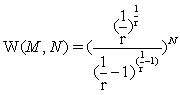 resulting in both cases to an exponential increase of the microstates with N and thus extensivity of the Boltzmann entropy since S=kBlnW. It is thus the Stirling approximation that is extensive in nature within the context used here and not the Boltzmann entropy.
resulting in both cases to an exponential increase of the microstates with N and thus extensivity of the Boltzmann entropy since S=kBlnW. It is thus the Stirling approximation that is extensive in nature within the context used here and not the Boltzmann entropy.
A comment on text book examples that show the extensive nature of the Boltzmann entropy
Many text books on physical chemistry and statistical mechanics show by using the examples given above (such as the ideal gas and the lattice gas models) the extensive character of the Boltzmann entropy by using the Stirling approximation of equation 1. As demonstrated above, the Stirling formula of equation 1 can however not be used for the thermodynamic limit because it is infinitely wrong for N→ ∞ (i.e., limN→∞(lnN! – NlnN + N)= ∞; Figure 1). Furthermore, by applying an extended Stirling formula (i.e., equation 5), that approximates lnN! at the thermodynamic limit, lack of the extensivity of the Boltzmann entropy is obtained. It is thus recommended to omit in text books derivations that show the extensive nature of the Boltzmann entropy with examples having indistinguishable particles.
The Boltzmann entropy enables a perpetum mobile
Ruelle in his rigorous approach on statistical mechanics approaches the study of the Boltzmann entropy without the use of the Stirling formula. Adjusted to the context used here and the examples given above with the presence of two subsystems (i.e., boxes), which are independent of each others, he finds that the Boltzmann entropy is subadditive
 (41)
(41)
In line with the examples above, the equality sign is deleted yielding S1+2>S1+S2 for a N particles system.
With this inequality, in the following the Boltzmann entropy as a description of the thermodynamic entropy will be put ad absurdum. For this it is assumed that the Boltzmann entropy describes its thermodynamics counterpart including equation 41:
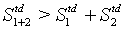 (42)
(42)
This inequality equation can now be used to construct a perpetum mobile of second order: Let us start with a single box filled with ideal gas molecules at a given pressure p and temperature T in thermodynamic equilibrium. The box is now divided into two independent boxes of equal size, the same pressure p, and temperature T. Both boxes are still in a thermodynamic equilibrium. However, the sum of the entropy of the two subsystems decreased somewhat because of the inequality equation 42 and thus the system is able to do work described by a positive change in the Gibbs free energy although the system is in the thermodynamic equilibrium (note, this statement is of course absurd since a system in equilibrium is not able to do work). Next, the two boxes are put together. Since they do not differ in their temperature T, pressure p and density 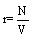 , nothing happens when the two are put together resulting in the starting state in its thermodynamic equilibrium. With this two steps process a cyclic machine can be constructed that is able to perform work from nothing, which is a perpetum mobile. Obviously, this is not possible as stated by the second law of thermodynamics indicating again that the Boltzmann entropy is not representing its thermodynamic counterpart.
, nothing happens when the two are put together resulting in the starting state in its thermodynamic equilibrium. With this two steps process a cyclic machine can be constructed that is able to perform work from nothing, which is a perpetum mobile. Obviously, this is not possible as stated by the second law of thermodynamics indicating again that the Boltzmann entropy is not representing its thermodynamic counterpart.
Alternative counting of micro-states to rescue the Boltzmann entropy
Under the request for a strict extensive entropy function and based on the mathematical analysis above either an alternative microscopic description of the thermodynamic entropy is required or the counting of micro-states including the normalization N!, introduced by Gibbs, which accounts for the indistinguishability of the particles, must be altered. Let us first consider the latter case. In light of the extensive nature of the Stirling approximation a normalization that would result in an extensive Boltzmann entropy would be NNe-bn with b being a constant (Table 1). In the most simple case with b=0, the normalization would be NN. Following this suggestion, for the ideal gas example introduced above the number of possible micro-states would then be given by 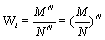 . A physical meaning of this entity is that the number of micro-states is given by the volume that a single particle is accommodating to the power of N. With other words, the probability of a particle p to be at site M is given by the particle density
. A physical meaning of this entity is that the number of micro-states is given by the volume that a single particle is accommodating to the power of N. With other words, the probability of a particle p to be at site M is given by the particle density 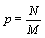 . This normalization results in an extensive Boltzmann entropy for the ideal gas example given above (Table 1). Furthermore, the Boltzmann entropy of a fully occupied ideal gas with M=N is 0 as one would expect, which is actually not the case of the Boltzmann entropy calculated by the use of equation 7. Using this new normalization, extensivity of the Boltzmann entropy is also obtained for the examples Boltzmann Entropy enables a perpetum mobile (Table 1) and Alternative counting of micro-states to rescue the Boltzmann Entropy, if they are diluted systems (i.e., M >>N). However, while this mathematically driven normalization appears to be physically more or less sound for the given examples in other systems the factorial N! attributed in general to the number of permutations makes sense, is prominent, and can not be put aside easily.
. This normalization results in an extensive Boltzmann entropy for the ideal gas example given above (Table 1). Furthermore, the Boltzmann entropy of a fully occupied ideal gas with M=N is 0 as one would expect, which is actually not the case of the Boltzmann entropy calculated by the use of equation 7. Using this new normalization, extensivity of the Boltzmann entropy is also obtained for the examples Boltzmann Entropy enables a perpetum mobile (Table 1) and Alternative counting of micro-states to rescue the Boltzmann Entropy, if they are diluted systems (i.e., M >>N). However, while this mathematically driven normalization appears to be physically more or less sound for the given examples in other systems the factorial N! attributed in general to the number of permutations makes sense, is prominent, and can not be put aside easily.
An alternative to the Boltzmann entropy
In the request for an alternative microscopic description of the thermodynamic entropy we would like to refer the reader to the recently introduced concept by Riek suggesting that entropy is a consequence of a postulated discreteness of time [16]. By introducing a quantum time an arrow of time is introduced already at the microscopic physics level (as already mentioned by Pauli within a different context [22]) through a friction term in the discrete Newton’s equation. This yields a term in the total energy of the system defined as the microscopic entropy. Interestingly, its ensemble average approximates the Boltzmann entropy at thermodynamic equilibrium. Thus, it connects the microscopic physics with the macroscopic one without a statistical argument. It is extensive in nature and thus resolves the problems discussed here.
The presented calculations show that for the ideal gas and other simple text book examples the Boltzmann entropy is not extensive unless the generally used Stirling approximation is used. Since the extensive nature of entropy is an important request of thermodynamics as otherwise the second law of thermodynamics is violated, the claim of statistical mechanics that the Boltzmann entropy is a microscopic description of its thermodynamic analog is therefore challenged. This odd observation suggests to find alternative microscopy descriptions of entropy such as the microscopy entropy introduced by Riek [18], or an alternative counting of micro states within the frame work of the Boltzmann entropy as suggested here. It is stimulating to realize that the concept of entropy appears to be still puzzling albeit its amazing success in thermodynamics and statistical mechanics.